Alea iacta est
“Everybody knows that the dice are loaded", everybody rolls with their fingers crossed…” Leonard Cohen
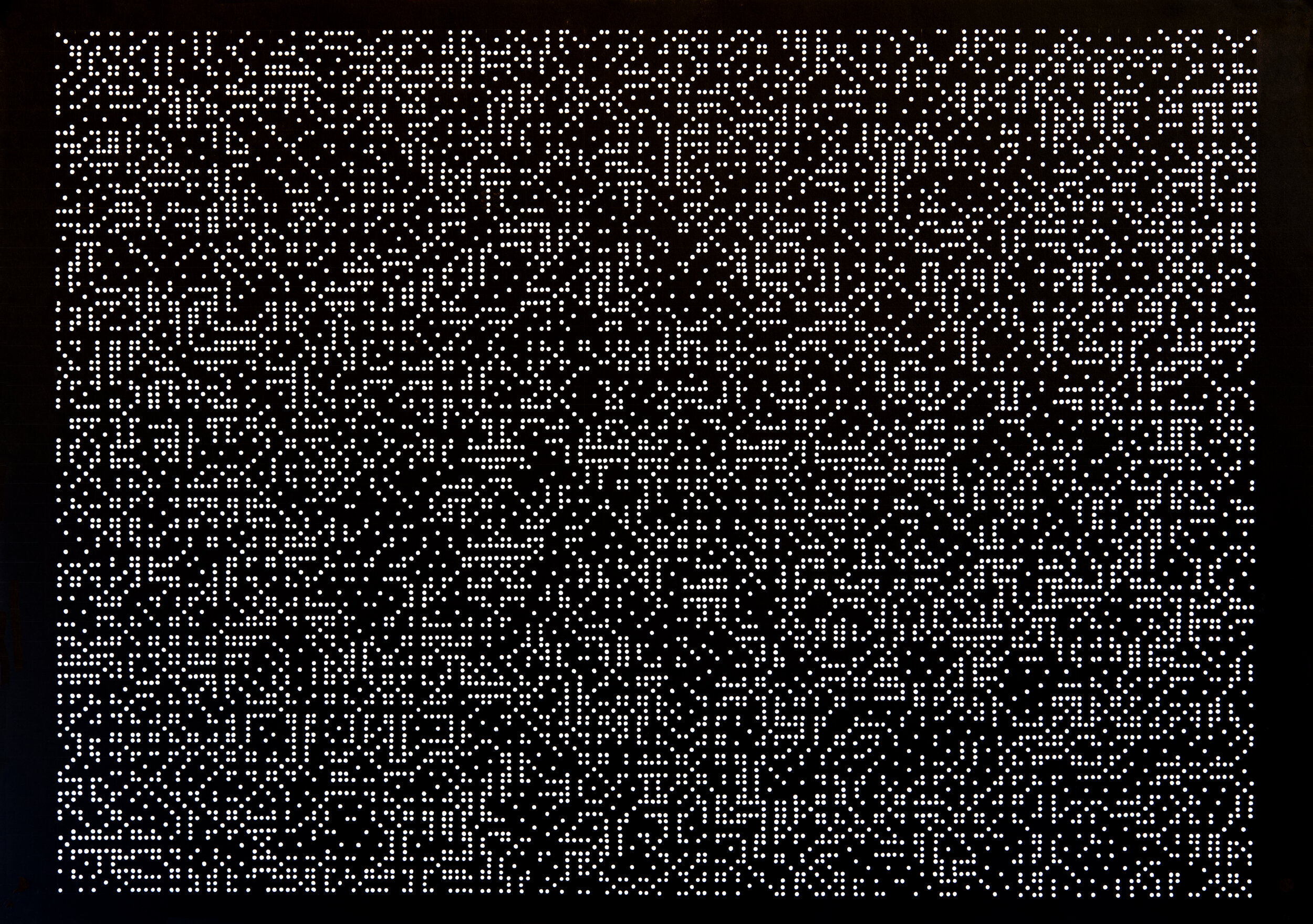

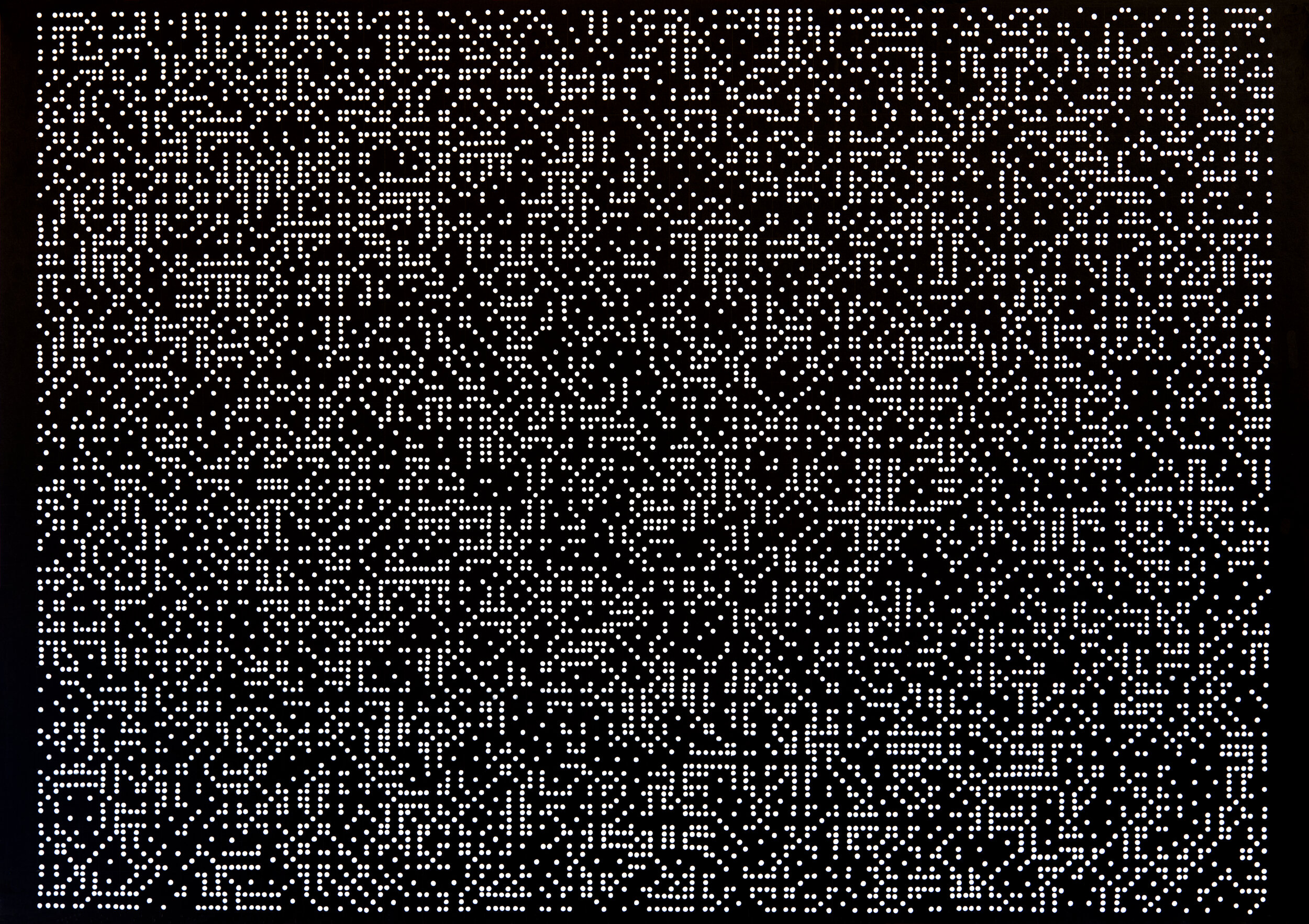
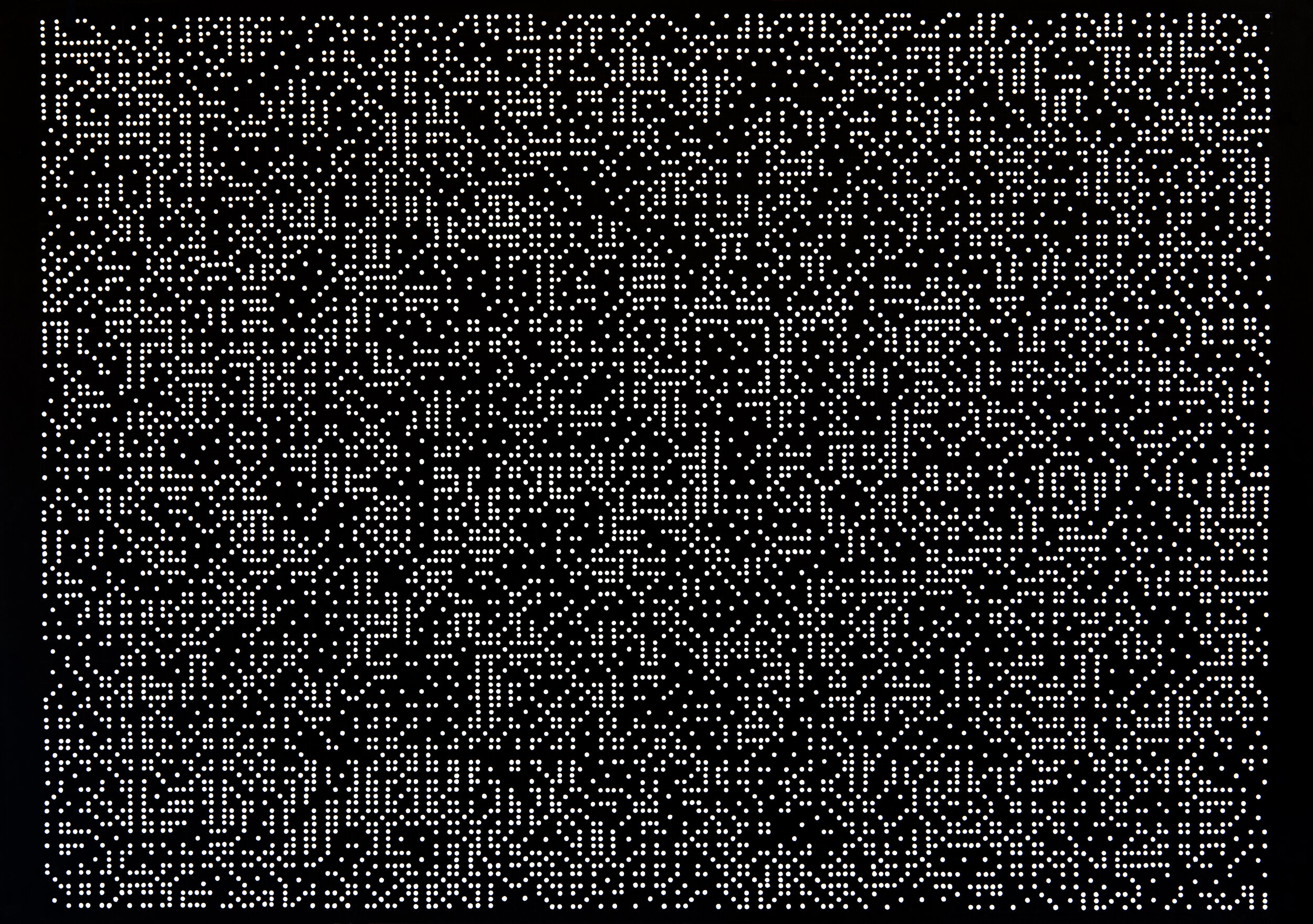
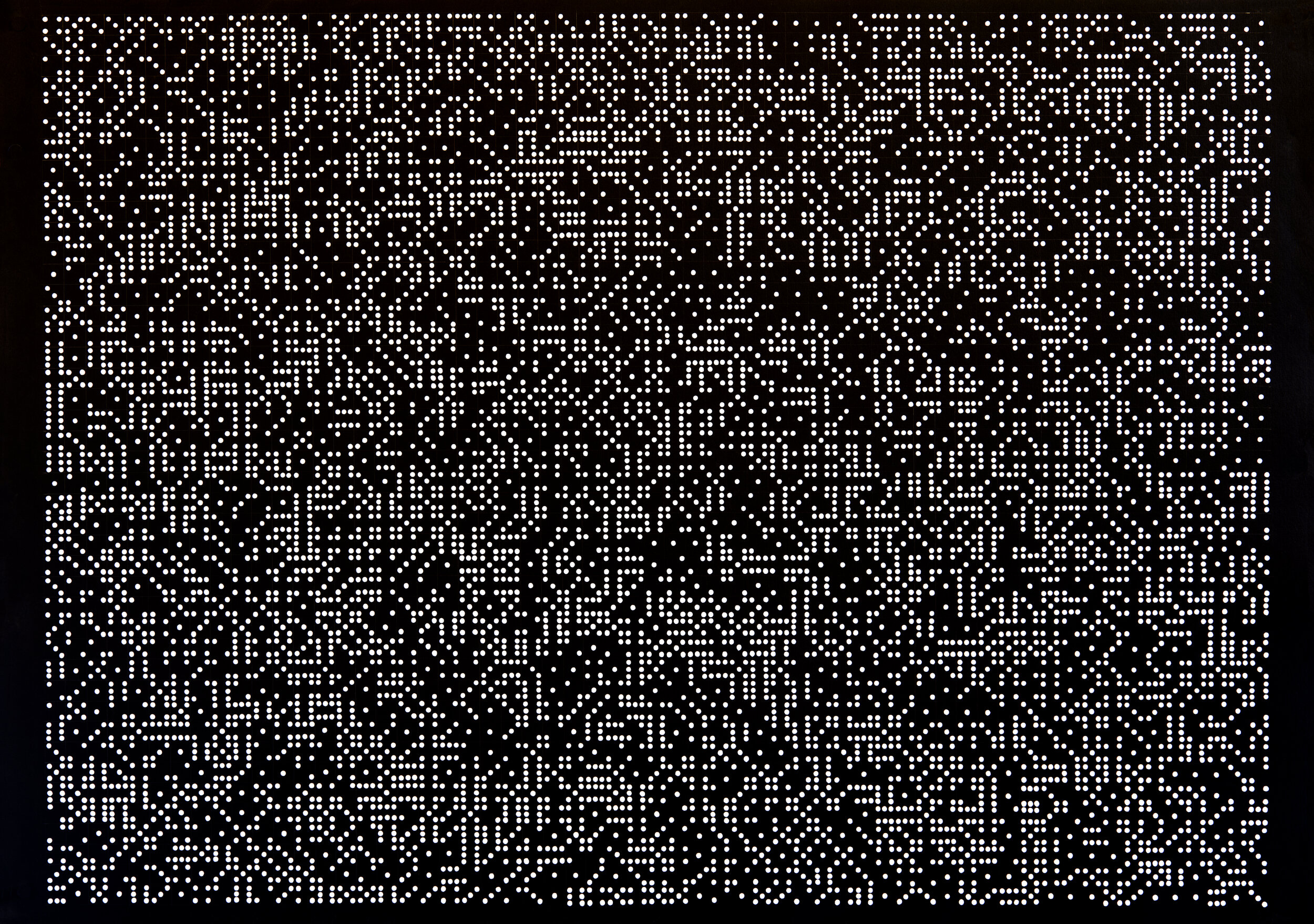
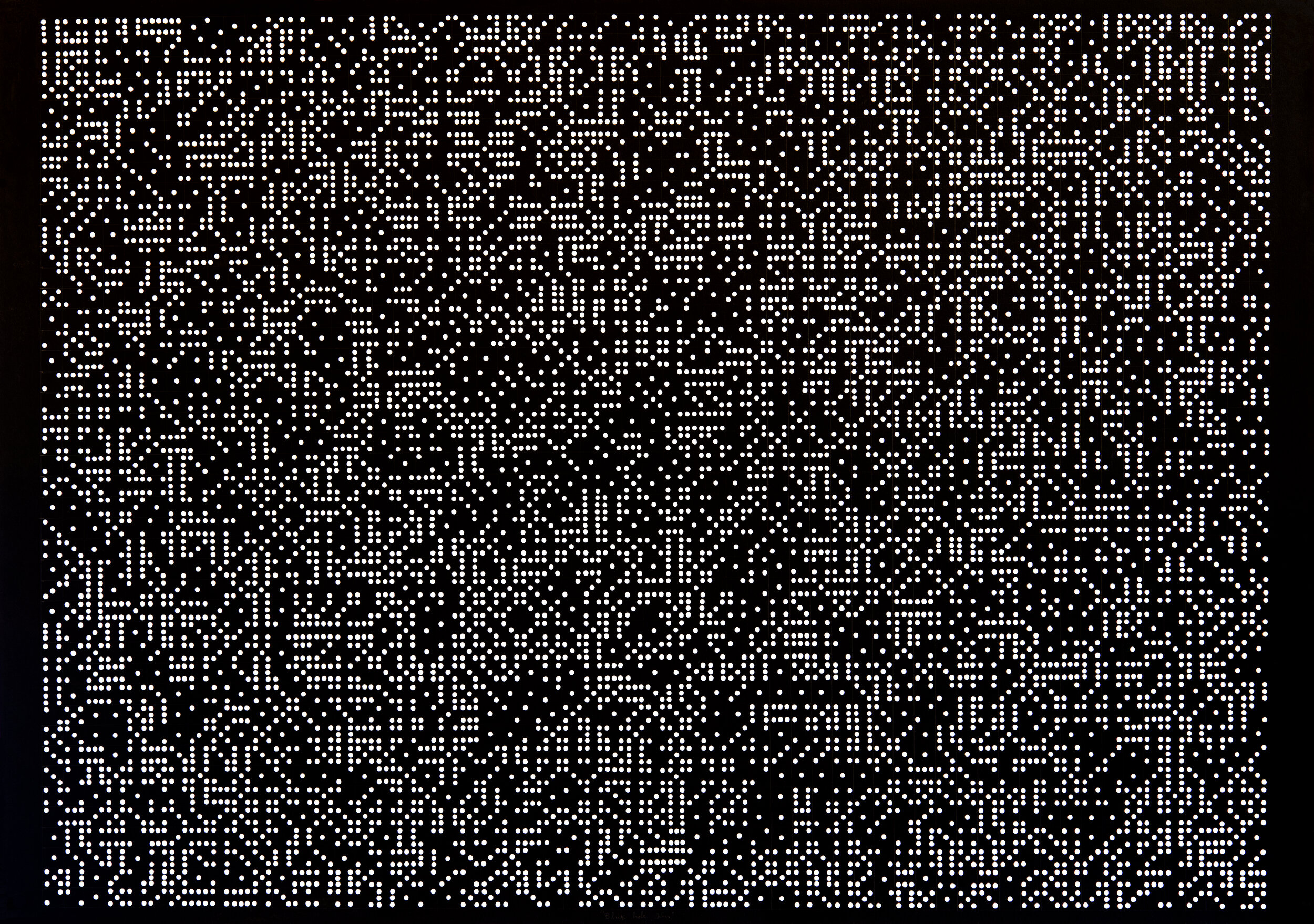
Dice Drawings made by rolling a different six sided die for each drawing. Ink and graphite pencil on paper. 30X 40 INCHES.
WE ARE FREE TO CONNECT THE DOTS ANY WAY WE WANT TO!


A whimsical random dice waltz with AI
How does Artificial intelligence understand chance and it’s nature?
Chat GPT VERSION 3.5 wa invited to play a game: “Imagine you are rolling a six sided die. Tell me the result of the dice roll.”" We repeated this until completing this drawing made by this collaboration and interaction between CHAT GPT V3.5 and Myself. Now its there for us to see the results and try to understand how its definition of chance, its method to simulate it, and to compare it with the results we get by rolling physical dice.
“The virtual charming whimsical charming dice waltz of uncertainty” Drawing made by simulating dice results with CHAT GPT Version 3.5. Ink and graphite pencil on paper. 30 x40 inches.
ARE THERE TWO EXACT DICE?
I Love dice! Why is it for?
Dice are beautiful as an example of good design. My bet is that an alien intelligence would understand their concept faster than many other artifacts they could find if they would land in our beloved and endangered planet. And they would love them as I do! Their form and functionality match so well, that we don’t need too much explanation to use them, and to understand the complex, interesting and metaphysical question they are addressing: is “randomness” real? Or it’s just a word to disguise our incapability to fully explain events from a logical and rational point of view.
Dice are designed to be rolled, so once they land on a surface and stop moving, we can look at their upper surface and get a message. A message that can be in the form of one dot, two dots, three dots, four dots, five dots or six dots. The idea is that we will only know the message, once the die is cast. In theory, this is impossible to know for certain, but this is questionable. According to the general idea of probability, you could get close to make a prediction, based on enough collected data, obtained from rolling the same dice in a similar way of rolling it. But there’s also a concept of each dice roll being a discrete event, meaning that it won't be determined by a previous roll or result. Even if there is a tendency in the die, each roll of the ide is an independent event. There are a lot of subtleties around this problem, that I’m excited to explore. I don’t want to believe blindly in the authority of probability, science or religion. I want to let the dice speak for themselves! And to create a dialogue with the concepts around it that come from probability, religion, physics, metaphysics, mathematics, speculation, and philosophy, among other possible ways of thinking.
How “random” dice are? How random can they be? How can I test them and observe how they are creating what we call “random” results? Can we understand enough to be able to predict the next roll? Can a computer learn how to do this? If so, how accurate can it be? Would it beat us on a gambling session? These are practical questions we can address using technology like electronics, computers and off course, dice! (Which could arguably or poetically be understood as an analog, electricity-less computer or machine).
People, including me, take the word “randomness” for granted. When I roll a die I do it assuming it will give me an unpredictable or “random” outcome. This also happens with the use of computers. And when we use our phone or music player in the Shuffle mode. We want to believe the randomness they produce is “perfect”. But according to Donald Knuth:
“Many random number generators in use today are not very good. There is a tendency for people to avoid learning anything about such subroutines; quite often we find that some old method that is comparatively unsatisfactory has blindly been passed down from one programmer to another, and today’s users have no understanding of its limitations.”
— Donald Knuth; The Art of Computer Programming, Volume 2.
This series of drawings are the starting point of Slow numbers. A project about the nature of randomness, using different methods of “random generation”.
As today, those methods are physical dice, computer algorithms that simulate random outcomes(digital dices), and human simulated dices (people pretending to be dice).
The idea is to also explore alternative new ways to generate what we call random outcomes.
Please visit Slow numbers to see more.
How many drawings will I have to do to make two exact ones?
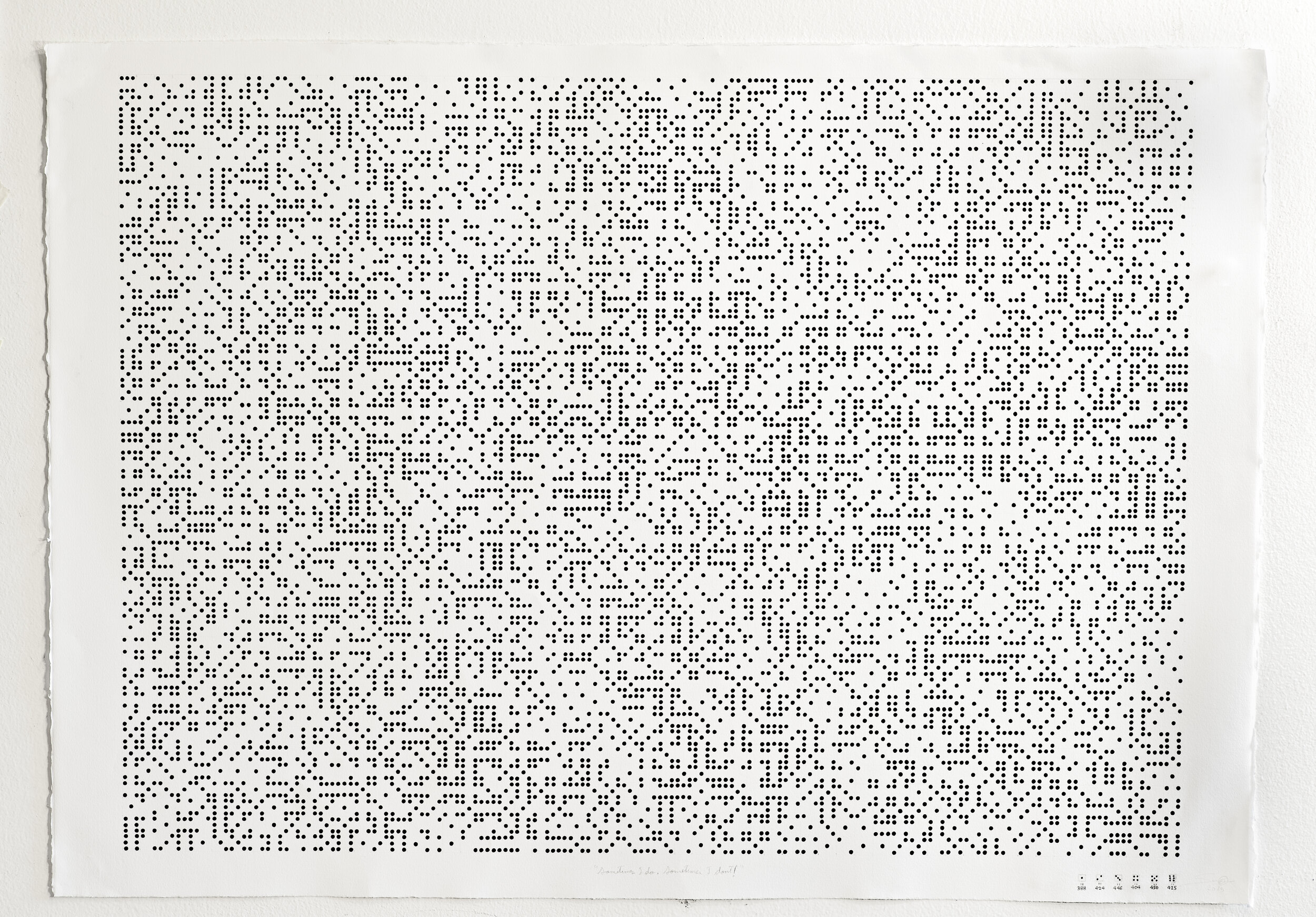


“Sometimes I do, sometimes I don’t” Dice Drawing made by rolling a different six sided die for each drawing. Ink and graphite pencil on paper. 30X 40 INCHES.
ALEA IACTA EST DIGITAL INTERACTIVE VERSION (Do it yourself)
If you feel like drawing, you can make your own by clicking the mouse or hitting enter, to roll the ide.
If you are not as patient as me, you can just hit enter to get your dice drawing in a more efficient way…
This interactive drawing uses the digital random algorithm to generate these masterpieces. How loaded the digital die is?
Roll it and find out for yourself!
Designed and coded by the one and only Daniel Castaño. A very talented Interactive and graphic designer, creative technologist and Engineer, and artist. Thank you Dani!
https://trafalmejo.github.io/data-visualization/howrandom/
To see Dani’s work please visit:


